Cents and Ratios

Cents
Cents are a unit of measurement used in music to describe the difference in pitch between two notes. One cent is equal to 1/100th of a semitone. This means that there are 100 cents in a semitone, and 1200 cents in an octave.
The concept of cents allows musicians and audio engineers to communicate and describe differences in pitch with high precision. To the untrained ear, differences of around 25 cents are noticeable. With training, musicians can learn to detect 2 cent or even 1 cent differences.
Do the maths
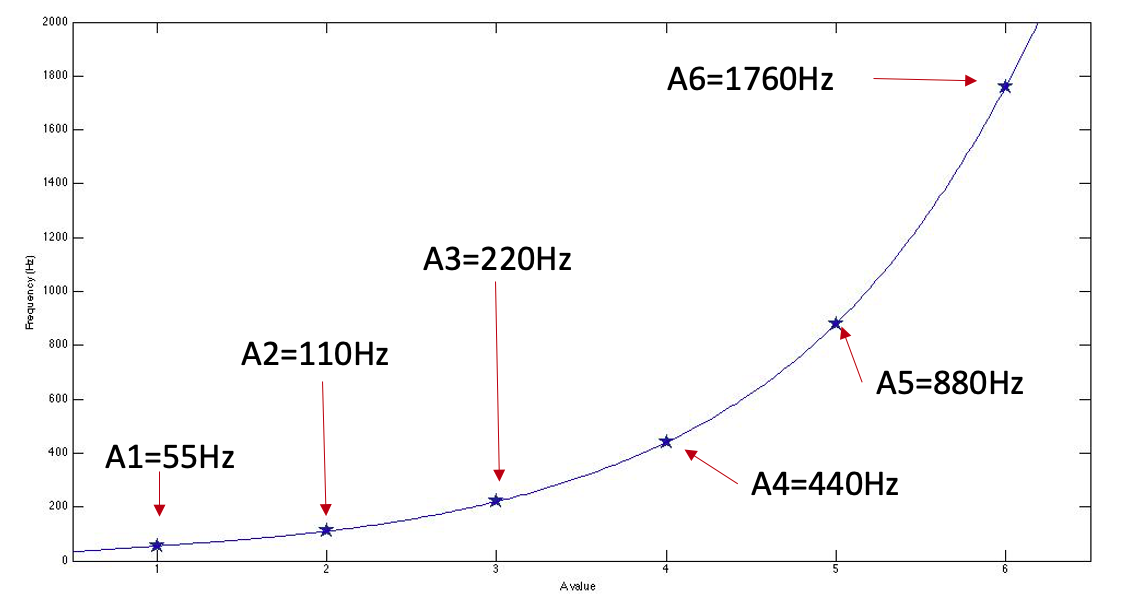
In standard "concert" tuning the A4 note has a frequency of 440 Hz. If you want to find out the frequency of the note two semitones above, B4, which is 200 cents higher, you can calculate the frequency using the following formula:
New frequency = Original frequency * 2^(Cents difference / 1200)
(The hat ^ symbol means "to the power of")
In this case, the calculation would be:
B4 frequency = 440 * 2^(200 / 1200) ≈ 493.88 Hz
Using this formula you can calculate the frequency of a note from another note, as long as you know the frequency of one of the notes and how many semitones apart they are.
For example
C4 frequency = 440 * 2^(300 / 1200) ≈ 523.25 Hz
D4 frequency = 440 * 2^(400 / 1200) ≈ 554.37 Hz
And so on.
Similarly, if you want to calculate the frequency of smaller shifts, for example half a semitone, also known as a quartertone, which is sometimes used to approximate the scales used in Arabic and Middle Eastern music:
50 cents above A4 = 440 * 2^(50 / 1200) ≈ 452.89 Hz
One thing to note is that the frequency difference between the same number of cents changes as you move up and down, because it is logarithmic, meaning the differences are larger for lower tones and smaller for higher tones.
Ratios
Ratios are the building blocks of Just Intonation scales, a tuning system that emphasizes harmonically pure intervals by basing them on simple whole number relationships. Rooted in the natural harmonic series, Just Intonation creates consonant and pleasing sounds that resonate with our innate perception of harmony. In contrast to equal temperament, which is the standard tuning system for most Western music, Just Intonation prioritizes the purity of intervals over the ability to easily modulate between keys.
In Just Intonation, each note's frequency is determined by a specific ratio relative to a fundamental frequency or tonic. These ratios create a variety of intervals, ranging from the most consonant, like unisons (1:1) and octaves (2:1), to more complex and dissonant intervals. By using these simple whole number ratios, Just Intonation scales enable musicians to explore a unique tonal landscape that is distinct from the equally tempered 12-tone system.
While Just Intonation offers a rich harmonic palette and a closer connection to the natural harmonic series, it also presents challenges for musicians who wish to modulate between keys or play with fixed-pitch instruments, such as pianos or keyboards. Nonetheless, the exploration of Just Intonation scales provides a fascinating insight into the world of tuning and opens up new possibilities for musical expression and experimentation.
- Unison (1:1) - This ratio represents the same note played together. The frequencies are identical, creating a perfect unison.
- Octave (2:1) - In this ratio, the higher note's frequency is double that of the lower note. This interval is found in most tuning systems and divides the frequency spectrum into equal parts, creating a sense of balance.
- Perfect Fifth (3:2) - This ratio means the higher note's frequency is 1.5 times that of the lower note. The perfect fifth is considered one of the most consonant and stable intervals in music.
- Perfect Fourth (4:3) - With this ratio, the higher note's frequency is 1.333 times that of the lower note. The perfect fourth is another consonant interval, often used as a building block for harmony.
- Major Third (5:4) - This ratio indicates that the higher note's frequency is 1.25 times that of the lower note. The major third is a sweet-sounding interval and a fundamental component of major chords.
- Minor Third (6:5) - In this ratio, the higher note's frequency is 1.2 times that of the lower note. The minor third is a fundamental component of minor chords and has a somewhat melancholic or introspective quality.